Crystal Field Examples¶

from CrystalField import CrystalField, CrystalFieldFit

data_ws1 = Load('NdOs2Al10_5K35meV_Ecut_0to3ang_bp15V1.xye')

# Set up the crystal field model.
cf = CrystalField('Nd', 'C2v', Temperature=5, FWHM=1,
    B20=0.19, B22=0.11, B40=-0.0004, B42=-0.002, B44=-0.012, B60=5.e-05, B62=0.00054, B64=-0.0006, B66=0.0008)
cf.PeakShape = 'Lorentzian'
cf.IntensityScaling = 2   # Scale factor if data is not in absolute units (mbarn/sr/f.u./meV), will be fitted.

# Runs the fit
fit = CrystalFieldFit(Model=cf, InputWorkspace=data_ws1,MaxIterations=200)
fit.fit()

# Plots the data and print fitted parameters
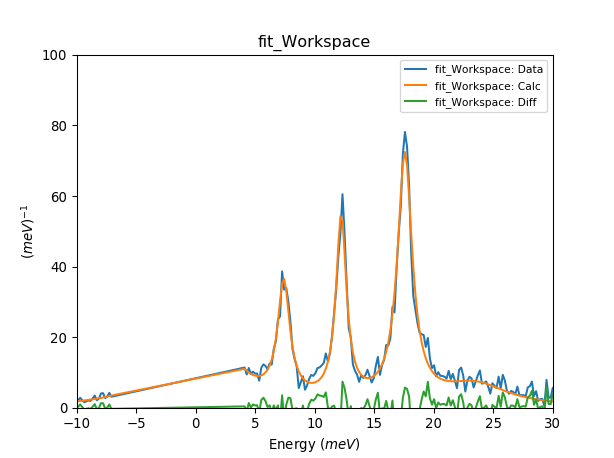
plotSpectrum('fit_Workspace',[0,1,2],error_bars=False)
plt.xlim([-10,30])
plt.ylim([0,100])

for parnames in ['B20', 'B22','B40','B42', 'B44', 'B60','B62','B64','B66']:
    print (parnames+' = '+str(fit.model[parnames])+' meV')

table = mtd['fit_Parameters']
print ('Cost function value = '+str(table.row(table.rowCount()-1)['Value']))

from CrystalField import CrystalField, CrystalFieldFit, Background, Function, ResolutionModel
from mantidqtinterfaces.PyChop import PyChop2

# load the data
data_ws1 = Load('MER38435_10p22meV.txt')
data_ws2 = Load('MER38436_10p22meV.txt')

# Sets up a resolution function
merlin = PyChop2('MERLIN', 'G', 250.)
merlin.setEi(10.)
resmod = ResolutionModel(merlin.getResolution, xstart=-10, xend=9.0, accuracy=0.01)

Kelvin_to_meV = 1./11.6

# Parameters from https://doi.org/10.1016/0921-4526(91)90575-Y
lit_par = {'B20': -.27, 'B22': -.34, 'B40':1e-6, 'B42':0.04158, 'B44':0.01369, 'B60':0.112e-3, 'B62':0.4185e-3, 'B64':-0.555e-3, 'B66':0.588e-3} # K
for parameter in lit_par.keys():
   lit_par[parameter] *= Kelvin_to_meV

# Set up the crystal field model.
cf = CrystalField('Ho', 'D2', Temperature=[25, 50], FWHM=0.3, **lit_par)
cf.PeakShape = 'Lorentzian'
cf.IntensityScaling = [0.2, 0.2]   # Scale factor if data is not in absolute units (mbarn/sr/f.u./meV), will be fitted.
cf.ResolutionModel = [resmod, resmod]
cf.background = Background(peak=Function('Gaussian', Height=1200, Sigma=0.4/2.3))
cf.background[1].peak.ties(Height=1400, PeakCentre=0, Sigma=0.4/2.3)

# Runs the fit
fit = CrystalFieldFit(Model=cf, InputWorkspace=[data_ws1, data_ws2], MaxIterations=100, Output='Fit')
fit.fit()

# Plots the fit
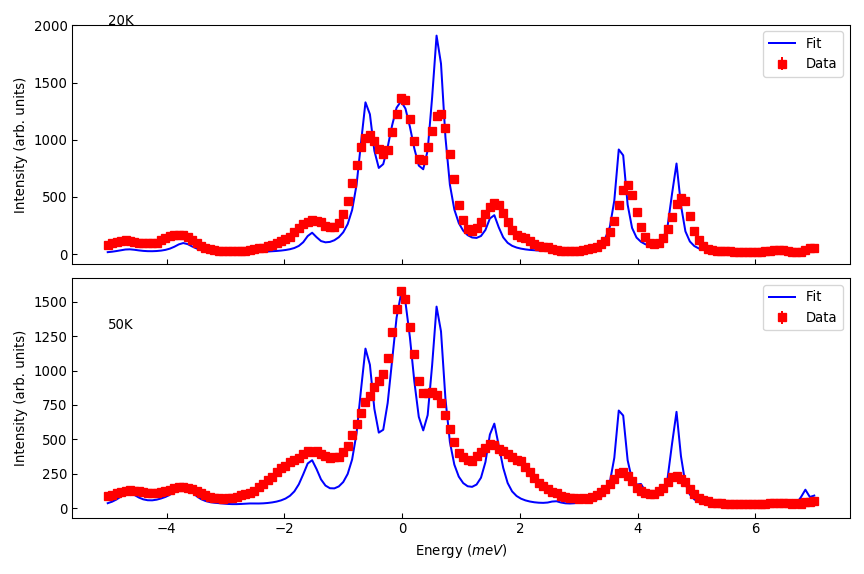
res_ws = [mtd['Fit_Workspace_0'], mtd['Fit_Workspace_1']]
titles = ['20K', '50K']
titley = [2000, 1300]
fig, axs = plt.subplots(figsize=(9, 6), nrows=2, ncols=1, sharex=True, subplot_kw={'projection':'mantid'})
for ii in range(2):
    axs[ii].errorbar(res_ws[ii], 'rs', wkspIndex=0, label='Data')
    axs[ii].plot(res_ws[ii], 'b-', wkspIndex=1, label='Fit')
    axs[ii].legend()
    axs[ii].set_ylabel('Intensity (arb. units)')
    axs[ii].tick_params(axis='both', direction='in')
    axs[ii].annotate(titles[ii], (-5, titley[ii]))
axs[0].set_xlabel('')
fig.tight_layout()
fig.show()

from CrystalField import CrystalField, CrystalFieldFit, PhysicalProperties
import matplotlib.pyplot as plt

sus_a = Load('NdOs2Al10_sus_a.txt')
sus_b = Load('NdOs2Al10_sus_b.txt')
sus_c = Load('NdOs2Al10_sus_c.txt')

cf = CrystalField('Nd', 'C2v',
     B20=0.19, B22=0.11, B40=-0.0004, B42=-0.002, B44=-0.012, B60=5.e-05, B62=0.00054, B64=-0.0006, B66=0.0008)

# Simultaneously fit data measured in a, b and c directions
cf.PhysicalProperty = [
     PhysicalProperties('susc', Hdir=[1,0,0], Inverse=True, Unit='cgs'),
     PhysicalProperties('susc', Hdir=[0,1,0], Inverse=True, Unit='cgs'),
     PhysicalProperties('susc', Hdir=[0,0,1], Inverse=True, Unit='cgs')]

fit = CrystalFieldFit(Model=cf, InputWorkspace=[sus_a, sus_b, sus_c], MaxIterations=100, Output='fit_susc')
fit.fit()

# Print fitted parameters and plot results
blm={}
for parname in ['B20','B22', 'B40', 'B42', 'B44','B60','B62','B64','B66']:
    blm[parname] = cf[parname]
    print parname+"="+str(cf[parname])
calc_a = mtd['fit_susc_Workspaces'][0]
calc_b = mtd['fit_susc_Workspaces'][1]
calc_c = mtd['fit_susc_Workspaces'][2]
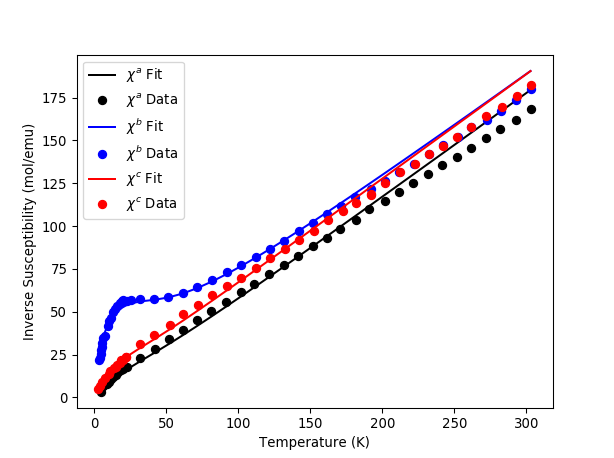
plt.plot(calc_a.readX(1),calc_a.readY(1),'-k',label='$\chi^a$ Fit')
plt.plot(mtd['sus_a'].readX(0),mtd['sus_a'].readY(0),'ok',label='$\chi^a$ Data')
plt.plot(calc_b.readX(1),calc_b.readY(1),'-b',label='$\chi^b$ Fit')
plt.plot(mtd['sus_b'].readX(0),mtd['sus_b'].readY(0),'ob',label='$\chi^b$ Data')
plt.plot(calc_c.readX(1),calc_c.readY(1),'-r',label='$\chi^c$ Fit')
plt.plot(mtd['sus_c'].readX(0),mtd['sus_c'].readY(0),'or',label='$\chi^c$ Data')
plt.legend(loc='upper left')
plt.xlabel('Temperature (K)')
plt.ylabel('Inverse Susceptibility (mol/emu)')
plt.show()

from CrystalField import CrystalField, CrystalFieldFit

datadir = ''
data_ws1=Load(datadir+'cecuga3Mlacuga3_15meV5K0to2p5angbp2V1.xye')
data_ws2=Load(datadir+'cecuga3Mlacuga3fp824_15meV50K0to2p5angbp2V1.xye')
data_ws3=Load(datadir+'cecuga3Mlacuga3fp824_15meV100K0to2p5angbp2V1.xye')

# Set up the crystal field model for multiple spectra.
# This is indicated by the number of elements in the list of temperatures.
# Optionally other parameters like FWHM and IntensityScaling can be lists if these initial parameters for each
#    spectra should differ.
cf = CrystalField('Ce', 'C4v', Temperature=[5,50,100], FWHM=[1,1,1], B20=0.0633, B40=0.01097, B44=0.09985)
cf.PeakShape = 'Lorentzian'
cf.IntensityScaling = [2, 2, 2]   # Scale factor if data is not in absolute units (mbarn/sr/f.u./meV), will be fitted.

# Runs the fit
fit = CrystalFieldFit(Model=cf, InputWorkspace=[data_ws1, data_ws2, data_ws3], MaxIterations=200)
fit.fit()

# Plots the data and print fitted parameters
plotSpectrum('fit_Workspace_0', [0,1,2], error_bars=False)
plt.ylim([0,20])
plt.xlim([-10,15])
plotSpectrum('fit_Workspace_1', [0,1,2], error_bars=False)
plt.ylim([0,20])
plt.xlim([-10,15])
plotSpectrum('fit_Workspace_2', [0,1,2], error_bars=False)
plt.ylim([0,20])
plt.xlim([-10,15])

# Prints output parameters and cost function.
for parnames in ['B20', 'B40','B44']:
    print (parnames+' = '+str(fit.model[parnames])+' meV')
table = mtd['fit_Parameters']
print ('Cost function value = '+str(table.row(table.rowCount()-1)['Value']))

B20 = 0.101723272944 meV
B40 = 0.012725904646 meV
B44 = 0.0890276949598 meV
Cost function value = 1.79652249577

from CrystalField import Background, CrystalField, Function

# Sets up the crystal field model
refpars = {'B20':0.2, 'B40':-0.00164, 'B60':0.0001146, 'B66':0.001509}

cf = CrystalField('Pr', 'C6v', Temperature=5, **refpars)
cf.IntensityScaling = 0.05
cf.FWHMVariation = 0.0
cf.PeakShape = 'Gaussian'

# Creates a background using a list of Function objects
cf.background = Background(functions=[Function('PseudoVoigt', Intensity=101, FWHM=0.8, Mixing=0.84, PeakCentre=-0.1),
                                      Function('Gaussian', Height=1.8, Sigma=0.27, PeakCentre=9.0)])

# Fixes all the parameters of the PseudoVoigt to their current values.
cf.background.functions[0].fix('all')

# Fixes the PeakCentre and Height of the Gaussian to their current values.
cf.background.functions[1].fix('PeakCentre', 'Height')

from CrystalField import Background, CrystalField, Function

# Sets up the crystal field model
refpars = {'B20':0.2, 'B40':-0.00164, 'B60':0.0001146, 'B66':0.001509}

cf = CrystalField('Pr', 'C6v', Temperature=5, **refpars)
cf.IntensityScaling = 0.05
cf.FWHMVariation = 0.0
cf.PeakShape = 'Gaussian'

# Creates a background using a list of Function objects
cf.background = Background(functions=[Function('PseudoVoigt', Intensity=101, FWHM=0.8, Mixing=0.84, PeakCentre=-0.1),
                                      Function('Gaussian', Height=1.8, Sigma=0.27, PeakCentre=9.0)])

# Ties some of the parameters in the Gaussian to different values.
cf.background.functions[1].ties(PeakCentre=9.0, Height=2.0)